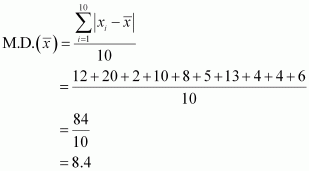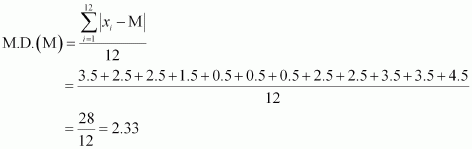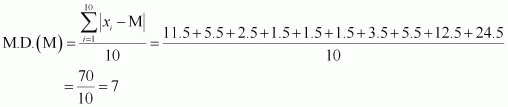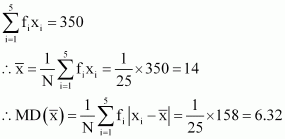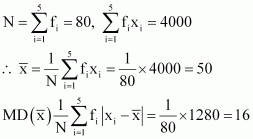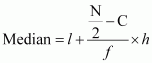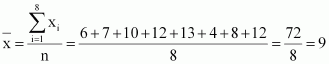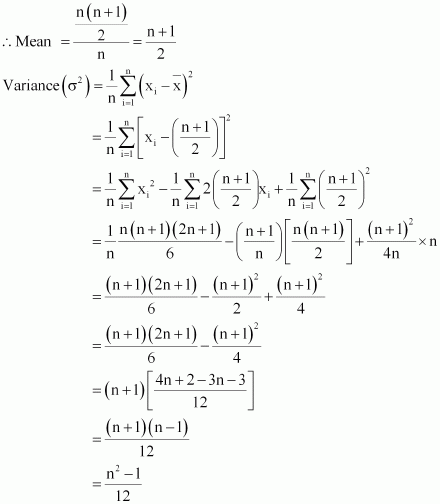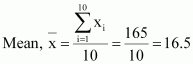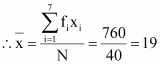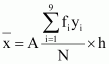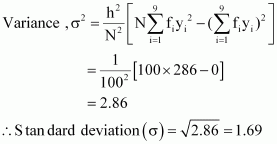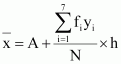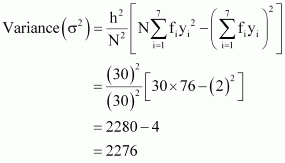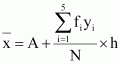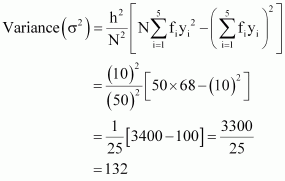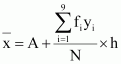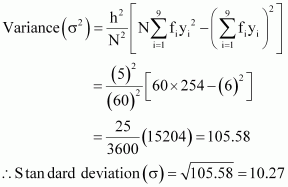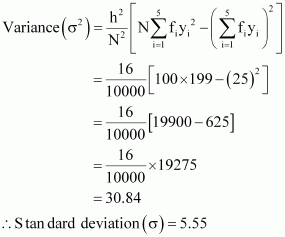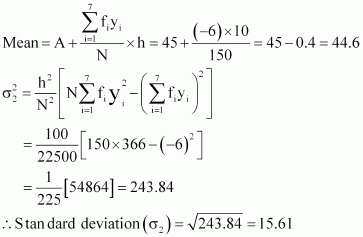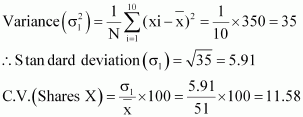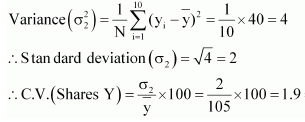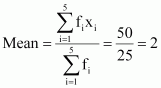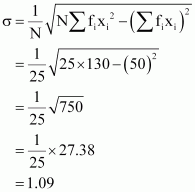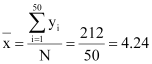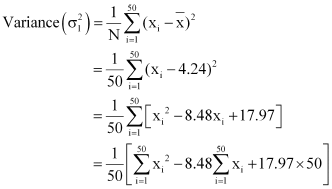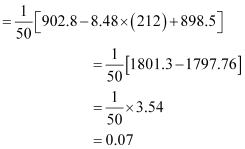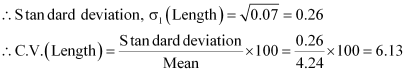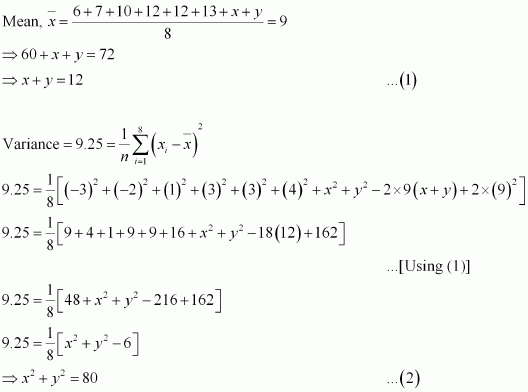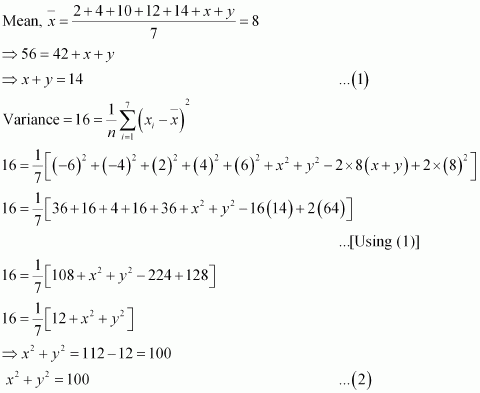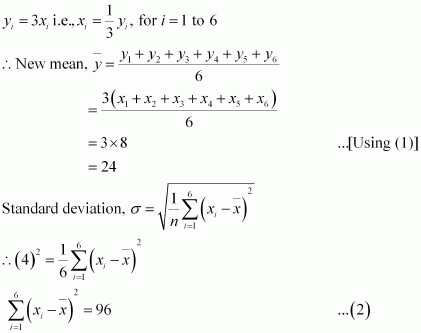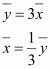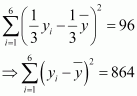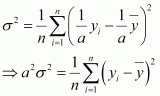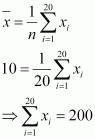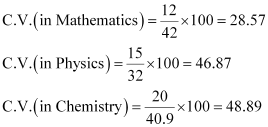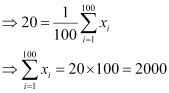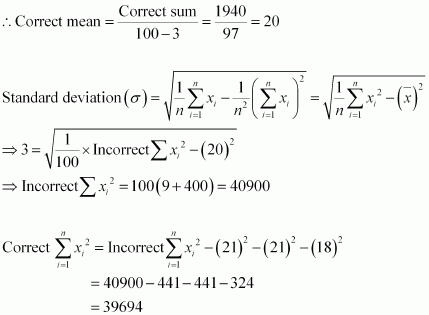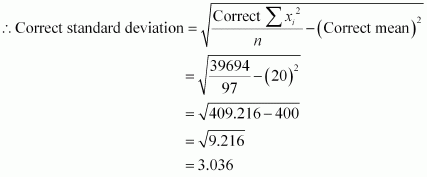NCERT Solutions for Class 11 Maths Chapter 15 – Statistics
Page No 360:
Question 1:
Find the mean deviation about the mean for the data
4, 7, 8, 9, 10, 12, 13, 17
ANSWER:
The given data is
4, 7, 8, 9, 10, 12, 13, 17
Mean of the data, ![]()
The deviations of the respective observations from the mean ![]() are
are
–6, – 3, –2, –1, 0, 2, 3, 7
The absolute values of the deviations, i.e.![]() , are
, are
6, 3, 2, 1, 0, 2, 3, 7
The required mean deviation about the mean is
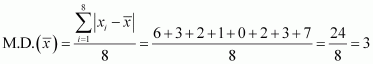
Page No 360:
Question 2:
Find the mean deviation about the mean for the data
38, 70, 48, 40, 42, 55, 63, 46, 54, 44
ANSWER:
The given data is
38, 70, 48, 40, 42, 55, 63, 46, 54, 44
Mean of the given data,
The deviations of the respective observations from the mean are
–12, 20, –2, –10, –8, 5, 13, –4, 4, –6
The absolute values of the deviations, i.e. , are
12, 20, 2, 10, 8, 5, 13, 4, 4, 6
The required mean deviation about the mean is
Page No 360:
Question 3:
Find the mean deviation about the median for the data.
13, 17, 16, 14, 11, 13, 10, 16, 11, 18, 12, 17
ANSWER:
The given data is
13, 17, 16, 14, 11, 13, 10, 16, 11, 18, 12, 17
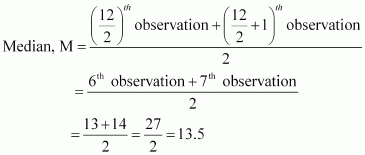
Here, the numbers of observations are 12, which is even.
Arranging the data in ascending order, we obtain
10, 11, 11, 12, 13, 13, 14, 16, 16, 17, 17, 18
The deviations of the respective observations from the median, i.e.are
–3.5, –2.5, –2.5, –1.5, –0.5, –0.5, 0.5, 2.5, 2.5, 3.5, 3.5, 4.5
The absolute values of the deviations,, are
3.5, 2.5, 2.5, 1.5, 0.5, 0.5, 0.5, 2.5, 2.5, 3.5, 3.5, 4.5
The required mean deviation about the median is
Page No 360:
Question 4:
Find the mean deviation about the median for the data
36, 72, 46, 42, 60, 45, 53, 46, 51, 49
ANSWER:
The given data is
36, 72, 46, 42, 60, 45, 53, 46, 51, 49
Here, the number of observations is 10, which is even.
Arranging the data in ascending order, we obtain
36, 42, 45, 46, 46, 49, 51, 53, 60, 72
The deviations of the respective observations from the median, i.e.are
–11.5, –5.5, –2.5, –1.5, –1.5, 1.5, 3.5, 5.5, 12.5, 24.5
The absolute values of the deviations,, are
11.5, 5.5, 2.5, 1.5, 1.5, 1.5, 3.5, 5.5, 12.5, 24.5
Thus, the required mean deviation about the median is
Page No 360:
Question 5:
Find the mean deviation about the mean for the data.
| xi | 5 | 10 | 15 | 20 | 25 |
| fi | 7 | 4 | 6 | 3 | 5 |
ANSWER:
-
xi
fi
fi xi
5
7
35
9
63
10
4
40
4
16
15
6
90
1
6
20
3
60
6
18
25
5
125
11
55
25
350
158
Page No 360:
Question 6:
Find the mean deviation about the mean for the data
| xi | 10 | 30 | 50 | 70 | 90 |
| fi | 4 | 24 | 28 | 16 | 8 |
ANSWER:
-
xi
fi
fi xi
10
4
40
40
160
30
24
720
20
480
50
28
1400
70
16
1120
20
320
90
8
720
40
320
80
4000
1280
Page No 360:
Question 7:
Find the mean deviation about the median for the data.
| xi | 5 | 7 | 9 | 10 | 12 | 15 |
| fi | 8 | 6 | 2 | 2 | 2 | 6 |
ANSWER:
The given observations are already in ascending order.
Adding a column corresponding to cumulative frequencies of the given data, we obtain the following table.
-
xi
fi
c.f.
5
8
8
7
6
14
9
2
16
10
2
18
12
2
20
15
6
26
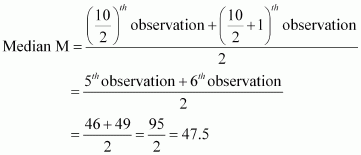
Here, N = 26, which is even.
Median is the mean of 13th and 14th observations. Both of these observations lie in the cumulative frequency 14, for which the corresponding observation is 7.
The absolute values of the deviations from median, i.e.are
-
|xi – M| 2
2
3
5
8
fi 8
6
2
2
2
6
fi |xi – M| 16
4
6
10
48
and
Page No 360:
Question 8:
Find the mean deviation about the median for the data
| xi | 15 | 21 | 27 | 30 | 35 |
| fi | 3 | 5 | 6 | 7 | 8 |
ANSWER:
The given observations are already in ascending order.
Adding a column corresponding to cumulative frequencies of the given data, we obtain the following table.
-
xi fi c.f.
15
3
3
21
5
8
27
6
14
30
7
21
35
8
29
Here, N = 29, which is odd.
observation = 15th observation
This observation lies in the cumulative frequency 21, for which the corresponding observation is 30.
∴ Median = 30
The absolute values of the deviations from median, i.e.are
-
|xi – M| 15
9
3
5
fi 3
5
6
7
8
fi |xi – M| 45
45
18
40
∴
Page No 361:
Question 9:
Find the mean deviation about the mean for the data.
| Income per day | Number of persons |
| 0-100 | 4 |
| 100-200 | 8 |
| 200-300 | 9 |
| 300-400 | 10 |
| 400-500 | 7 |
| 500-600 | 5 |
| 600-700 | 4 |
| 700-800 | 3 |
ANSWER:
The following table is formed.
-
Income per day
Number of persons fi
Mid-point xi
fi xi
0 – 100
4
50
200
308
1232
100 – 200
8
150
1200
208
1664
200 – 300
9
250
2250
108
972
300 – 400
10
350
3500
8
80
400 – 500
7
450
3150
92
644
500 – 600
5
550
2750
192
960
600 – 700
4
650
2600
292
1168
700 – 800
3
750
2250
392
1176
50
17900
7896
Here,
Page No 361:
Question 10:
Find the mean deviation about the mean for the data
| Height in cms | Number of boys |
| 95-105 | 9 |
| 105-115 | 13 |
| 115-125 | 26 |
| 125-135 | 30 |
| 135-145 | 12 |
| 145-155 | 10 |
ANSWER:
The following table is formed.
-
Height in cms
Number of boys fi
Mid-point xi
fi xi
95-105
9
100
900
25.3
227.7
105-115
13
110
1430
15.3
198.9
115-125
26
120
3120
5.3
137.8
125-135
30
130
3900
4.7
141
135-145
12
140
1680
14.7
176.4
145-155
10
150
1500
24.7
247
Here,
Page No 361:
Question 11:
Find the mean deviation about median for the following data:
| Marks | Number of girls |
| 0-10 | 6 |
| 10-20 | 8 |
| 20-30 | 14 |
| 30-40 | 16 |
| 40-50 | 4 |
| 50-60 | 2 |
ANSWER:
The following table is formed.
-
Marks
Number of girls fi
Cumulative frequency (c.f.)
Mid-point xi
|xi – Med.|
fi |xi – Med.|
0-10
6
6
5
22.85
137.1
10-20
8
14
15
12.85
102.8
20-30
14
28
25
2.85
39.9
30-40
16
44
35
7.15
114.4
40-50
4
48
45
17.15
68.6
50-60
2
50
55
27.15
54.3
50
517.1
The class interval containing theor 25th item is 20 – 30.
Therefore, 20 – 30 is the median class.
It is known that,
Here, l = 20, C = 14, f = 14, h = 10, and N = 50
∴ Median =
Thus, mean deviation about the median is given by,
Page No 361:
Question 12:
Calculate the mean deviation about median age for the age distribution of 100 persons given below:
| Age | Number |
| 16-20 | 5 |
| 21-25 | 6 |
| 26-30 | 12 |
| 31-35 | 14 |
| 36-40 | 26 |
| 41-45 | 12 |
| 46-50 | 16 |
| 51-55 | 9 |
ANSWER:
The given data is not continuous. Therefore, it has to be converted into continuous frequency distribution by subtracting 0.5 from the lower limit and adding 0.5 to the upper limit of each class interval.
The table is formed as follows.
-
Age
Number fi
Cumulative frequency (c.f.)
Mid-point xi
|xi – Med.|
fi |xi – Med.|
15.5-20.5
5
5
18
20
100
20.5-25.5
6
11
23
15
90
25.5-30.5
12
23
28
10
120
30.5-35.5
14
37
33
5
70
35.5-40.5
26
63
38
40.5-45.5
12
75
43
5
60
45.5-50.5
16
91
48
10
160
50.5-55.5
9
100
53
15
135
100
735
The class interval containing theor 50th item is 35.5 – 40.5.
Therefore, 35.5 – 40.5 is the median class.
It is known that,
Here, l = 35.5, C = 37, f = 26, h = 5, and N = 100
Thus, mean deviation about the median is given by,
Page No 371:
Question 1:
Find the mean and variance for the data 6, 7, 10, 12, 13, 4, 8, 12
ANSWER:
6, 7, 10, 12, 13, 4, 8, 12
Mean,
The following table is obtained.
-
xi
6
–3
9
7
–2
4
10
–1
1
12
3
9
13
4
16
4
–5
25
8
–1
1
12
3
9
74
Page No 371:
Question 2:
Find the mean and variance for the first n natural numbers
ANSWER:
The mean of first n natural numbers is calculated as follows.
Page No 371:
Question 3:
Find the mean and variance for the first 10 multiples of 3
ANSWER:
The first 10 multiples of 3 are
3, 6, 9, 12, 15, 18, 21, 24, 27, 30
Here, number of observations, n = 10
The following table is obtained.
-
xi
3
–13.5
182.25
6
–10.5
110.25
9
–7.5
56.25
12
–4.5
20.25
15
–1.5
2.25
18
1.5
2.25
21
4.5
20.25
24
7.5
56.25
27
10.5
110.25
30
13.5
182.25
742.5
Page No 371:
Question 4:
Find the mean and variance for the data
| xi | 6 | 10 | 14 | 18 | 24 | 28 | 30 |
| f i | 2 | 4 | 7 | 12 | 8 | 4 | 3 |
ANSWER:
The data is obtained in tabular form as follows.
-
xi
f i
fixi
6
2
12
–13
169
338
10
4
40
–9
81
324
14
7
98
–5
25
175
18
12
216
–1
1
12
24
8
192
5
25
200
28
4
112
9
81
324
30
3
90
11
121
363
40
760
1736
Here, N = 40,
Page No 371:
Question 5:
Find the mean and variance for the data
| xi | 92 | 93 | 97 | 98 | 102 | 104 | 109 |
| f i | 3 | 2 | 3 | 2 | 6 | 3 | 3 |
ANSWER:
The data is obtained in tabular form as follows.
-
xi
f i
fixi
92
3
276
–8
64
192
93
2
186
–7
49
98
97
3
291
–3
9
27
98
2
196
–2
4
8
102
6
612
2
4
24
104
3
312
4
16
48
109
3
327
9
81
243
22
2200
640
Here, N = 22,
Page No 371:
Question 6:
Find the mean and standard deviation using short-cut method.
| xi | 60 | 61 | 62 | 63 | 64 | 65 | 66 | 67 | 68 |
| fi | 2 | 1 | 12 | 29 | 25 | 12 | 10 | 4 | 5 |
ANSWER:
The data is obtained in tabular form as follows.
-
xi
fi
yi2
fiyi
fiyi2
60
2
–4
16
–8
32
61
1
–3
9
–3
9
62
12
–2
4
–24
48
63
29
–1
1
–29
29
64
25
65
12
1
1
12
12
66
10
2
4
20
40
67
4
3
9
12
36
68
5
4
16
20
80
100
220
286
Mean,
Page No 371:
Question 7:
Find the mean and variance for the following frequency distribution.
| Classes | 0-30 | 30-60 | 60-90 | 90-120 | 120-150 | 150-180 | 180-210 |
| Frequencies | 2 | 3 | 5 | 10 | 3 | 5 | 2 |
ANSWER:
-
Class
Frequency fi
Mid-point xi
yi2
fiyi
fiyi2
0-30
2
15
–3
9
–6
18
30-60
3
45
–2
4
–6
12
60-90
5
75
–1
1
–5
5
90-120
10
105
120-150
3
135
1
1
3
3
150-180
5
165
2
4
10
20
180-210
2
195
3
9
6
18
30
2
76
Mean,
Page No 372:
Question 8:
Find the mean and variance for the following frequency distribution.
| Classes | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 |
| Frequencies | 5 | 8 | 15 | 16 | 6 |
ANSWER:
| Class | Frequency fi | Mid-point xi | | yi2 | fiyi | fiyi2 |
| 0-10 | 5 | 5 | –2 | 4 | –10 | 20 |
| 10-20 | 8 | 15 | –1 | 1 | –8 | 8 |
| 20-30 | 15 | 25 | ||||
| 30-40 | 16 | 35 | 1 | 1 | 16 | 16 |
| 40-50 | 6 | 45 | 2 | 4 | 12 | 24 |
| 50 | 10 | 68 |
Mean,
Page No 372:
Question 9:
Find the mean, variance and standard deviation using short-cut method
| Height in cms | No. of children |
| 70-75 | 3 |
| 75-80 | 4 |
| 80-85 | 7 |
| 85-90 | 7 |
| 90-95 | 15 |
| 95-100 | 9 |
| 100-105 | 6 |
| 105-110 | 6 |
| 110-115 | 3 |
ANSWER:
| Class Interval | Frequency fi | Mid-point xi | | yi2 | fiyi | fiyi2 |
| 70-75 | 3 | 72.5 | –4 | 16 | –12 | 48 |
| 75-80 | 4 | 77.5 | –3 | 9 | –12 | 36 |
| 80-85 | 7 | 82.5 | –2 | 4 | –14 | 28 |
| 85-90 | 7 | 87.5 | –1 | 1 | –7 | 7 |
| 90-95 | 15 | 92.5 | ||||
| 95-100 | 9 | 97.5 | 1 | 1 | 9 | 9 |
| 100-105 | 6 | 102.5 | 2 | 4 | 12 | 24 |
| 105-110 | 6 | 107.5 | 3 | 9 | 18 | 54 |
| 110-115 | 3 | 112.5 | 4 | 16 | 12 | 48 |
| 60 | 6 | 254 |
Mean,
Page No 372:
Question 10:
The diameters of circles (in mm) drawn in a design are given below:
| Diameters | No. of children |
| 33-36 | 15 |
| 37-40 | 17 |
| 41-44 | 21 |
| 45-48 | 22 |
| 49-52 | 25 |
ANSWER:
| Class Interval | Frequency fi | Mid-point xi | | fi2 | fiyi | fiyi2 |
| 32.5-36.5 | 15 | 34.5 | –2 | 4 | –30 | 60 |
| 36.5-40.5 | 17 | 38.5 | –1 | 1 | –17 | 17 |
| 40.5-44.5 | 21 | 42.5 | ||||
| 44.5-48.5 | 22 | 46.5 | 1 | 1 | 22 | 22 |
| 48.5-52.5 | 25 | 50.5 | 2 | 4 | 50 | 100 |
| 100 | 25 | 199 |
Here, N = 100, h = 4
Let the assumed mean, A, be 42.5.
Mean,
Page No 375:
Question 1:
From the data given below state which group is more variable, A or B?
| Marks | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 |
| Group A | 9 | 17 | 32 | 33 | 40 | 10 | 9 |
| Group B | 10 | 20 | 30 | 25 | 43 | 15 | 7 |
ANSWER:
Firstly, the standard deviation of group A is calculated as follows.
| Marks | Group A fi | Mid-point xi | | yi2 | fiyi | fiyi2 |
| 10-20 | 9 | 15 | –3 | 9 | –27 | 81 |
| 20-30 | 17 | 25 | –2 | 4 | –34 | 68 |
| 30-40 | 32 | 35 | –1 | 1 | –32 | 32 |
| 40-50 | 33 | 45 | ||||
| 50-60 | 40 | 55 | 1 | 1 | 40 | 40 |
| 60-70 | 10 | 65 | 2 | 4 | 20 | 40 |
| 70-80 | 9 | 75 | 3 | 9 | 27 | 81 |
| 150 | –6 | 342 |
Here, h = 10, N = 150, A = 45
The standard deviation of group B is calculated as follows.
| Marks | Group B fi | Mid-point xi | | yi2 | fiyi | fiyi2 |
| 10-20 | 10 | 15 | –3 | 9 | –30 | 90 |
| 20-30 | 20 | 25 | –2 | 4 | –40 | 80 |
| 30-40 | 30 | 35 | –1 | 1 | –30 | 30 |
| 40-50 | 25 | 45 | ||||
| 50-60 | 43 | 55 | 1 | 1 | 43 | 43 |
| 60-70 | 15 | 65 | 2 | 4 | 30 | 60 |
| 70-80 | 7 | 75 | 3 | 9 | 21 | 63 |
| 150 | –6 | 366 |
Since the mean of both the groups is same, the group with greater standard deviation will be more variable.
Thus, group B has more variability in the marks.
Page No 375:
Question 2:
From the prices of shares X and Y below, find out which is more stable in value:
| X | 35 | 54 | 52 | 53 | 56 | 58 | 52 | 50 | 51 | 49 |
| Y | 108 | 107 | 105 | 105 | 106 | 107 | 104 | 103 | 104 | 101 |
ANSWER:
The prices of the shares X are
35, 54, 52, 53, 56, 58, 52, 50, 51, 49
Here, the number of observations, N = 10
The following table is obtained corresponding to shares X.
| xi | | |
| 35 | –16 | 256 |
| 54 | 3 | 9 |
| 52 | 1 | 1 |
| 53 | 2 | 4 |
| 56 | 5 | 25 |
| 58 | 7 | 49 |
| 52 | 1 | 1 |
| 50 | –1 | 1 |
| 51 | ||
| 49 | –2 | 4 |
| 350 |
The prices of share Y are
108, 107, 105, 105, 106, 107, 104, 103, 104, 101
The following table is obtained corresponding to shares Y.
| yi | | |
| 108 | 3 | 9 |
| 107 | 2 | 4 |
| 105 | ||
| 105 | ||
| 106 | 1 | 1 |
| 107 | 2 | 4 |
| 104 | –1 | 1 |
| 103 | –2 | 4 |
| 104 | –1 | 1 |
| 101 | –4 | 16 |
| 40 |
C.V. of prices of shares X is greater than the C.V. of prices of shares Y.
Thus, the prices of shares Y are more stable than the prices of shares X.
Page No 375:
Question 3:
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results:
| Firm A | Firm B | |
| No. of wage earners | 586 | 648 |
| Mean of monthly wages | Rs 5253 | Rs 5253 |
| Variance of the distribution of wages | 100 | 121 |
(i) Which firm A or B pays larger amount as monthly wages?
(ii) Which firm, A or B, shows greater variability in individual wages?
ANSWER:
(i) Monthly wages of firm A = Rs 5253
Number of wage earners in firm A = 586
∴Total amount paid = Rs 5253 × 586
Monthly wages of firm B = Rs 5253
Number of wage earners in firm B = 648
∴Total amount paid = Rs 5253 × 648
Thus, firm B pays the larger amount as monthly wages as the number of wage earners in firm B are more than the number of wage earners in firm A.
(ii) Variance of the distribution of wages in firm A = 100
∴ Standard deviation of the distribution of wages in firm
A ((σ1) =
Variance of the distribution of wages in firm = 121
∴ Standard deviation of the distribution of wages in firm
The mean of monthly wages of both the firms is same i.e., 5253. Therefore, the firm with greater standard deviation will have more variability.
Thus, firm B has greater variability in the individual wages.
Page No 376:
Question 4:
The following is the record of goals scored by team A in a football session:
| No. of goals scored | 1 | 2 | 3 | 4 | |
| No. of matches | 1 | 9 | 7 | 5 | 3 |
For the team B, mean number of goals scored per match was 2 with a standard
deviation 1.25 goals. Find which team may be considered more consistent?
ANSWER:
The mean and the standard deviation of goals scored by team A are calculated as follows.
| No. of goals scored | No. of matches | fixi | xi2 | fixi2 |
| 1 | ||||
| 1 | 9 | 9 | 1 | 9 |
| 2 | 7 | 14 | 4 | 28 |
| 3 | 5 | 15 | 9 | 45 |
| 4 | 3 | 12 | 16 | 48 |
| 25 | 50 | 130 |
Thus, the mean of both the teams is same.
The standard deviation of team B is 1.25 goals.
The average number of goals scored by both the teams is same i.e., 2. Therefore, the team with lower standard deviation will be more consistent.
Thus, team A is more consistent than team B.
Page No 376:
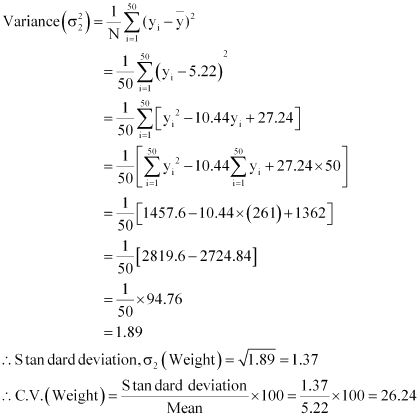
Question 5:
The sum and sum of squares corresponding to length x (in cm) and weight y
(in gm) of 50 plant products are given below:
Which is more varying, the length or weight?
ANSWER:
Here, N = 50
∴ Mean,
Mean,
Thus, C.V. of weights is greater than the C.V. of lengths. Therefore, weights vary more than the lengths.
Page No 380:
Question 1:
The mean and variance of eight observations are 9 and 9.25, respectively. If six of the observations are 6, 7, 10, 12, 12 and 13, find the remaining two observations.
ANSWER:
Let the remaining two observations be x and y.
Therefore, the observations are 6, 7, 10, 12, 12, 13, x, y.
From (1), we obtain
x2 + y2 + 2xy = 144 …(3)
From (2) and (3), we obtain
2xy = 64 … (4)
Subtracting (4) from (2), we obtain
x2 + y2 – 2xy = 80 – 64 = 16
⇒ x – y = ± 4 … (5)
Therefore, from (1) and (5), we obtain
x = 8 and y = 4, when x – y = 4
x = 4 and y = 8, when x – y = –4
Thus, the remaining observations are 4 and 8.
Page No 380:
Question 2:
The mean and variance of 7 observations are 8 and 16, respectively. If five of the observations are 2, 4, 10, 12 and 14. Find the remaining two observations.
ANSWER:
Let the remaining two observations be x and y.
The observations are 2, 4, 10, 12, 14, x, y.
From (1), we obtain
x2 + y2 + 2xy = 196 … (3)
From (2) and (3), we obtain
2xy = 196 – 100
⇒ 2xy = 96 … (4)
Subtracting (4) from (2), we obtain
x2 + y2 – 2xy = 100 – 96
⇒ (x – y)2 = 4
⇒ x – y = ± 2 … (5)
Therefore, from (1) and (5), we obtain
x = 8 and y = 6 when x – y = 2
x = 6 and y = 8 when x – y = – 2
Thus, the remaining observations are 6 and 8.
Page No 380:
Question 3:
The mean and standard deviation of six observations are 8 and 4, respectively. If each observation is multiplied by 3, find the new mean and new standard deviation of the resulting observations.
ANSWER:
Let the observations be x1, x2, x3, x4, x5, and x6.
It is given that mean is 8 and standard deviation is 4.
If each observation is multiplied by 3 and the resulting observations are yi, then
From (1) and (2), it can be observed that,
Substituting the values of xi and in (2), we obtain
Therefore, variance of new observations =
Hence, the standard deviation of new observations is
Page No 380:
Question 4:
Given that is the mean and σ2 is the variance of n observations x1, x2 … xn. Prove that the mean and variance of the observations ax1, ax2, ax3 …axn are
and a2 σ2, respectively (a ≠ 0).
ANSWER:
The given n observations are x1, x2 … xn.
Mean =
Variance = σ2
If each observation is multiplied by a and the new observations are yi, then
Therefore, mean of the observations, ax1, ax2 … axn, is .
Substituting the values of xiand in (1), we obtain
Thus, the variance of the observations, ax1, ax2 … axn, is a2 σ2.
Page No 380:
Question 5:
The mean and standard deviation of 20 observations are found to be 10 and 2, respectively. On rechecking, it was found that an observation 8 was incorrect. Calculate the correct mean and standard deviation in each of the following cases:
(i) If wrong item is omitted.
(ii) If it is replaced by 12.
ANSWER:
(i) Number of observations (n) = 20
Incorrect mean = 10
Incorrect standard deviation = 2
That is, incorrect sum of observations = 200
Correct sum of observations = 200 – 8 = 192
∴ Correct mean
Standard deviation, σ = 1n∑ni=1xi2 − 1n2(∑ni=1xi)2−−−−−−−−−−−−−−−−−−−−−−√
⇒2 = 1n∑ni=1xi2 − (1n∑ni=1xi)2−−−−−−−−−−−−−−−−−−−−−−√
⇒2 = 1n∑ni=1xi2 − (x¯)2−−−−−−−−−−−−−−−√[as, 1n∑ni=1x = x ]
⇒2 = 120×Incorrect∑ni=1xi2 − (10)2−−−−−−−−−−−−−−−−−−−−−−−−−−√
⇒4 = 120×Incorrect∑ni=1xi2 − 100⇒120×Incorrect∑ni=1xi2 = 104
⇒Incorrect∑ni=1xi2 = 2080Now, correct∑ni=1xi2 = Incorrect∑ni=1xi2 − (8)2
⇒correct∑ni=1xi2 = 2080 − 64 = 2016
∴ correct Standard Deviation = 1ncorrect∑ni=1xi2 − (correct mean)2−−−−−−√
⇒correct Standard Deviation = 119×2016 − (19219)2−−−−−−−−−−−−−−−−√
⇒correct Standard Deviation = 201619−(19219)2−−−−−−−−−−−√
⇒correct Standard Deviation = 1440√19 = 1210√19
⇒correct Standard Deviation = 12 × 3.16219 = 1.997Standard deviation, σ = 1n∑i=1nxi2 – 1n2∑i=1nxi2
⇒2 = 1n∑i=1nxi2 – 1n∑i=1nxi2⇒2 = 1n∑i=1nxi2 – x¯2 as, 1n∑i=1nx = x ⇒2 = 120×Incorrect∑i=1nxi2 – 102
⇒4 = 120×Incorrect∑i=1nxi2 – 100⇒120×Incorrect∑i=1nxi2 = 104
⇒Incorrect∑i=1nxi2 = 2080Now, correct∑i=1nxi2 = Incorrect∑i=1nxi2 – 82
⇒correct∑i=1nxi2 = 2080 – 64 = 2016∴ correct Standard Deviation = 1ncorrect∑i=1nxi2 – correct mean2
⇒correct Standard Deviation = 119×2016 – 192192
⇒correct Standard Deviation = 201619-192192
⇒correct Standard Deviation = 144019 = 121019
⇒correct Standard Deviation = 12 × 3.16219 = 1.997
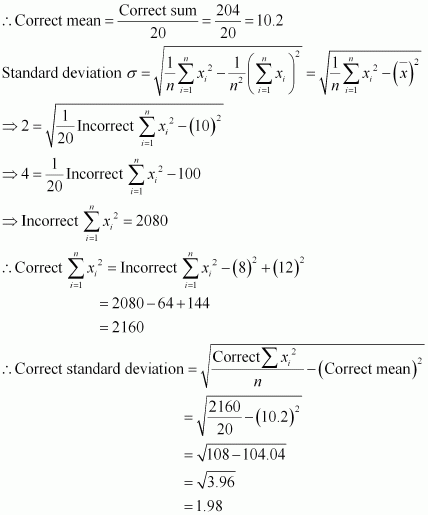
(ii) When 8 is replaced by 12,
Incorrect sum of observations = 200
∴ Correct sum of observations = 200 – 8 + 12 = 204
Page No 380:
Question 6:
The mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, Physics and Chemistry are given below:
| Subject | Mathematics | Physics | Chemistry |
| Mean | 42 | 32 | 40.9 |
| Standard deviation | 12 | 15 | 20 |
Which of the three subjects shows the highest variability in marks and which shows the lowest?
ANSWER:
Standard deviation of Mathematics = 12
Standard deviation of Physics = 15
Standard deviation of Chemistry = 20
The coefficient of variation (C.V.) is given by .
The subject with greater C.V. is more variable than others.
Therefore, the highest variability in marks is in Chemistry and the lowest variability in marks is in Mathematics.
Page No 380:
Question 7:
The mean and standard deviation of a group of 100 observations were found to be 20 and 3, respectively. Later on it was found that three observations were incorrect, which were recorded as 21, 21 and 18. Find the mean and standard deviation if the incorrect observations are omitted.
ANSWER:
Number of observations (n) = 100
Incorrect mean
Incorrect standard deviation
∴ Incorrect sum of observations = 2000
⇒ Correct sum of observations = 2000 – 21 – 21 – 18 = 2000 – 60 = 1940